objective Ques (161 results)
SSC CGL 201921)△ABC and △DBC are on the same BC but on opposite sides of it. AD and BC intersect each other at O.If AO = a cm, DO = b cm and the area of △ABC = x cm sq, then what is the area(in cm sq.) of △DBC ?
\({bx\over a}\)
SSC CGL 201922)In \(\triangle ABC\), the perpendiculars drawn from A, B and C meet the opposite sides at D, E and F, respectively. AD, BE and CF intersect at point P. If \(\angle EPD=116^0\) and the bisectors of \(\angle A\) and \(\angle B\) meet at Q, then the measure of is \(\angle AQB\) is :
\(122^0\)
SSC CGL 201923)The perimeters of two similar triangles ABC and PQR are 78 cm and 46.8 cm, respectively. If PQ = 11.7 cm., then the length of AB is:
19.5 cm.
SSC CGL 201924)In \(\triangle PQR\), I is the incentre of the triangle. If \(\angle QIR=107^0\), then what is the measure of \(\angle P\)?
\(34^0\)
SSC CGL 201925)The sides PQ and PR of \(\triangle PQR\) are produced to points S and T, respectively. The bisectors of \(\angle SQR\) and \(\angle TRQ\)meet at U. If \(\angle QUR=79^0\), then the measure of \(\angle P\) is:
\(22^0\)
SSC CGL 201926)In \(\triangle ABC\), AB = AC and D is a point on BC. If BD = 5 cm, AB = 12 cm and AD = 8 cm, then the length of CD is:
16 cm
SSC CGL 201927)The bisector of \(\angle B\) in \(\triangle ABC\) meets AC at D. If AB = 10 cm, BC = 11 cm and AC = 14 cm, then the length of AD is:
\(20\over3\) cm
SSC CGL 202028)Arrange the angles of the triangle from smallest to largest in the triangle, where the sides are AB = 7 cm, AC = 8 cm, and BC = 9 cm.
C,B,A
Measurement of angle is according to the length of opposite line.
So, The order of the angles of the triangle from smallest to largest in the triangle,
C < B < A.
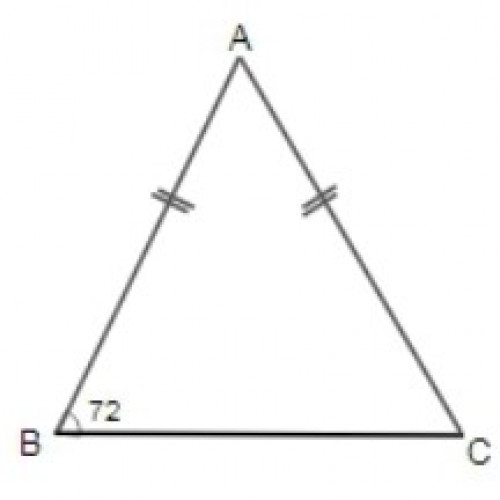
SSC CGL 202029)In the triangle, If AB = AC and \(\angle ABC = 72^0\), then \(\angle BAC\) is:
\(36^0\)
By triangle properties,
When AB = AC then \(\angle ABC = \angle ACB\) so,
In\(\triangle ABC,\)
\(\Rightarrow \angle ABC + \angle ACB + \angle BAC = 180^{0}\);
\(\Rightarrow \angle ABC + \angle ABC + \angle BAC = 180^{0}\);
\(\Rightarrow 72^{0} + 72^{0} + \angle BAC = 180^{0}\);
\(\Rightarrow \angle BAC = 180^{0} - 144^{0} = 36^{0}\)
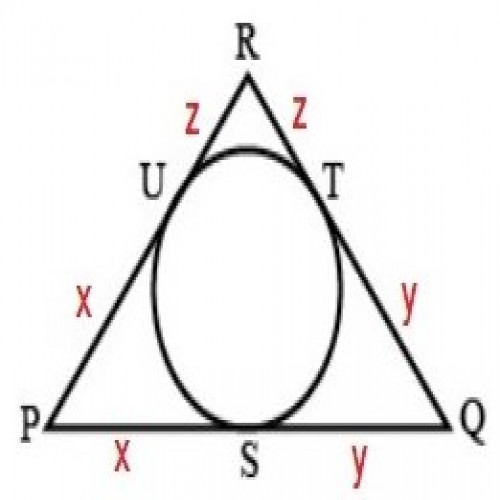
30)In the given figure, a circle inscribed in triangle PQR touches its sides PQ, QR and RP at points S, T and U,respectively. If PQ = 15 cm, QR= 10 cm, and RP = 12 cm, then find the lengths of PS, QT and RU?
SSC CGL 2020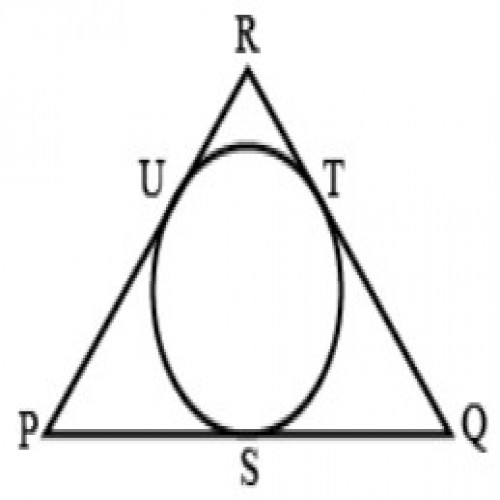
PS = 8.5 cm, QT = 6.5 cm and RU = 3.5 cm
PQ = 15 cm, QR= 10 cm, and RP = 12 cm. ;
PQ = x + y = 15------------------(i);
QR = y + z = 10----------(ii);
RP = z + x = 12 ------(iii);
Solving equation, we get :
z = 3.5 cm = RU;
y = 6.5 cm = QT;
x = 8.5 cm = PS